1 / 16
There are two gates. One goes to hell and the other goes to heaven. The gatekeeper asks a puzzle to Sahil in order to decide which gate he should open for him. Obviously if Sahil answers the puzzle correct , the gate to heaven will be opened else the gate to hell. According to the problem Sahil is given n consecutive integers from 1 to n ,which are written in a row. He has to put signs "+" and "-" in front of them so that the expression obtained is equal to 0 or, if the task is impossible to do,then Sahil should tell the gatekeeper “No solution exists for the given problem “.The gatekeeper expects Sahil to find his answer in minimum time using an efficient approach rather than examining all possible ways to place the signs. Sahil comes to you considering you as his friend. Would you help him out to get to the solution ?
2 / 16
There are n glasses on the table, all standing upside down. In one move, you are allowed to turn over exactly n 1 of them. Determine all values of n for which all the glasses can be turned up in the minimum number of moves.
3 / 16
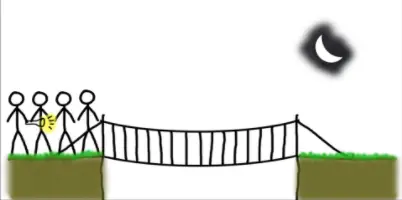
Four people need to cross a rickety bridge at night. Unfortunately, they have only one torch and the bridge is too dangerous to cross without one. The bridge is only strong enough to support two people at a time. Not all people take the same time to cross the bridge. Times for each person: 1 min, 2 mins, 7 mins and 10 mins. What is the shortest time needed for all four of them to cross the bridge?
4 / 16
A man has two ropes of varying thickness (Those two ropes are not identical, they aren’t the same density nor the same length nor the same width). Each rope burns in 60 minutes. He actually wants to measure 45 mins. How can he measure 45 mins using only these two ropes.
He can't cut the one rope in half because the ropes are non-homogeneous and he can’t be sure how long it will burn.
5 / 16
A certain town comprises of 100 married couples. Everyone in the town lives by the following rule: If a husband cheats on his wife, the husband is executed as soon as his wife reports about him to the mayor. No woman can report about other's husband. Every woman in the town knows about all the cheating husbands in the town except her own. One day, the mayor of the town announces to the whole town that there is at least 1 cheating husband in the town. How many days it will take to find out 19 Cheaters from the day of the announcement?
6 / 16
Connect 3 Houses with 3 Wells.
Connect 3 Houses with 3 Wells without the lines intersecting?

7 / 16
A Russian gangster kidnaps you. He puts two bullets in consecutive order in an empty six-round revolver, spins it, points it at your head and shoots. You're still alive. He then asks you, do you want me to spin it again and fire or pull the trigger again right away? For each option, what is the probability that you'll be shot?
8 / 16
You are given 8 identical looking balls. One of them is heavier than the rest of the 7 (all the others weigh exactly the same). You a provided with a simple mechanical balance and you are restricted to only 2 uses.
Find the heavier ball.
9 / 16
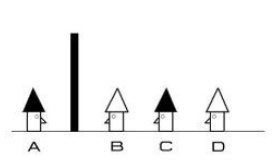
Shown above are four men buried up to their necks in the ground.
They can't move they can only look forward. Between A and B is a brick wall which is completely opaque (they absolutely can't see through it). They are all aware that each of them is wearing a hat, and that two of them are wearing a black hat, and two of them are wearing a white hat. They don't know what color THEY are wearing. However, each of them DOES know where the other three are buried.
In order to avoid being executed, one of them must call out to the executioner the color of their hat. If they get it wrong, everyone will be shot. They can't talk to each other and have only 10 minutes to figure it out.
After 60 seconds, one of them calls out.
QUESTION: Which one of them calls out? How can he be certain he knows the color of his hat?
10 / 16
You have 10 bags full of coins. In each bag are infinite coins. But one bag is full of forgeries, and you can’t remember which one. But you do know that genuine coins weigh 1 gram, but forgeries weigh 1.1 grams. You have to identify that bag in minimum readings. You are provided with a digital weighing machine.
Sapient Practice Set


